Unlocking The Mystery: How Many Sig Figs In 0.1?

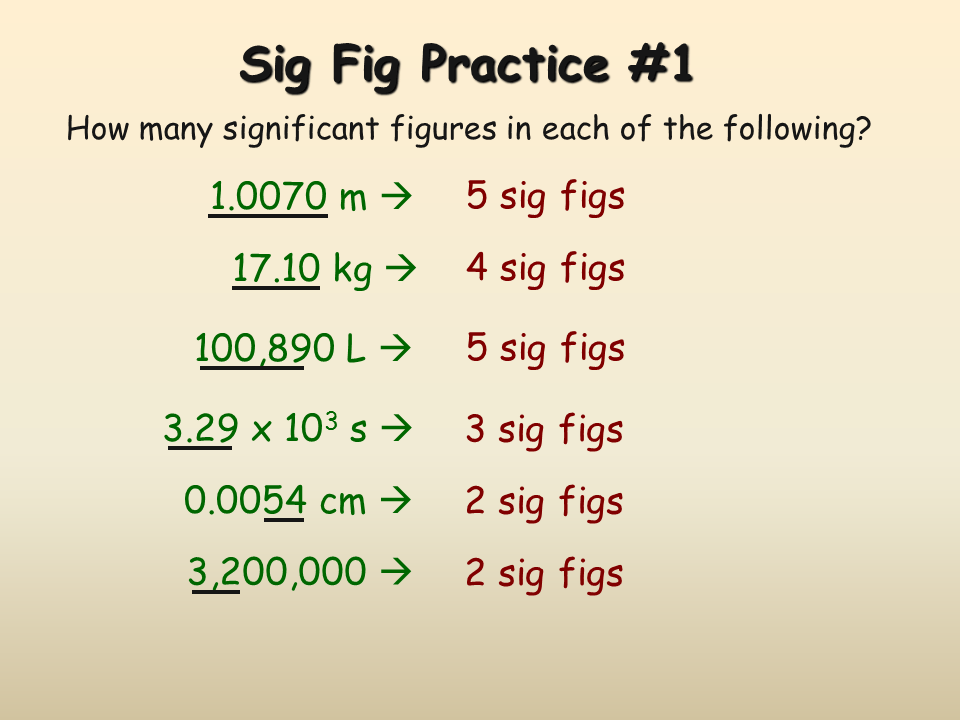
How many sig figs in 0.1? This question might seem straightforward at first glance, but it holds the key to understanding a fundamental concept in mathematics and science: significant figures. These are the digits that carry meaning contributing to a number's precision. Whether you're a student, a professional, or just someone curious about the world, knowing how to determine significant figures is crucial. In this article, we'll delve into the significance of significant figures, why they matter, and how they play a pivotal role in scientific measurements.

Significant figures, also known as sig figs, are a vital component in the world of mathematics, science, and engineering. They help us express numbers in a way that reflects their accuracy and precision. When dealing with measurements, it's essential to communicate the level of certainty that accompanies those measurements. Thus, understanding significant figures isn't just about counting digits; it's about acknowledging the precision of your data. In this article, we'll explore why this is important and how it can impact various fields.

Our journey into the world of significant figures will take us through the basics, offering insights into how sig figs work, why they matter, and how to apply them. We'll also tackle common misconceptions and frequently asked questions, ensuring that by the end of this article, you'll have a clear understanding of how many sig figs are in 0.1 and why it matters. So, buckle up as we embark on this informative and enlightening journey!

Table of Contents

• Understanding Significant Figures
• The Importance of Significant Figures in Measurements
• Determining Significant Figures
• Rules for Counting Significant Figures
• How Many Sig Figs in 0.1?
• Significant Figures in Scientific Notation
• Rounding and Significant Figures
• Significant Figures in Calculations
• Common Mistakes and Misconceptions
• Practical Applications of Significant Figures
• Significant Figures in Different Fields
• Real-World Examples
• Frequently Asked Questions
• Conclusion
• Further Reading and Resources

Understanding Significant Figures

Significant figures are a set of digits in a number that contribute to its precision. They are used in expressing numbers consistently in scientific and engineering contexts. A significant figure is any digit that is reliable and necessary to indicate the precision of a measurement. The concept of significant figures is crucial because it helps convey the uncertainty in measurements and calculations. This is vital as it prevents the communication of false precision and ensures that results are stated as precisely as the data allows.

The concept of significant figures revolves around the idea that not all digits in a number are equally important. For instance, in a measurement like 0.1, the "1" is significant, while the "0" is merely a placeholder. By understanding which digits are significant, we can accurately express and interpret data, ensuring that it communicates the right level of accuracy. This is especially important in scientific experiments and engineering projects where precision is paramount.

Significant figures also play a critical role in calculations. When performing mathematical operations, it's essential to maintain the integrity of the original data's precision. This ensures that any derived results are as accurate as the initial measurements allow. In the sections that follow, we'll delve deeper into how significant figures are determined and applied in various contexts.

The Importance of Significant Figures in Measurements

In the realm of scientific measurements, significant figures serve as a bridge between raw data and meaningful conclusions. Measurements are often subject to limitations due to the precision of the measuring instrument. Significant figures help indicate the reliability of these measurements by highlighting which digits are precise. This is crucial because it prevents the over-interpretation of data, ensuring that conclusions are drawn based on accurate information.

The importance of significant figures extends beyond just individual measurements. When measurements are used in calculations, the precision of the final result is determined by the least precise measurement. By adhering to the rules of significant figures, scientists and engineers can ensure that their results are as accurate as their data allows. This is essential for maintaining the integrity of scientific research and engineering projects.

In summary, significant figures are crucial for expressing the precision of measurements accurately. They provide a standardized way of indicating which digits in a number are reliable, preventing the misinterpretation of data. Understanding and applying the rules of significant figures is essential for anyone working in fields where precision and accuracy are paramount.

Determining Significant Figures

Determining the number of significant figures in a number is a straightforward process once you understand the basic rules. These rules help identify which digits in a number are significant and contribute to its precision. By following these guidelines, you can ensure that your measurements and calculations accurately reflect the precision of your data.

The first step in determining significant figures is to identify all non-zero digits as significant. These digits are always considered significant because they contribute to the precision of the number. For example, in the number 123.45, all five digits are significant because they are non-zero.

Next, any zeros between non-zero digits are also considered significant. These zeros serve as placeholders and indicate the precision of the measurement. For instance, in the number 1002, the zeros are significant because they fall between non-zero digits.

Leading zeros, or zeros that appear before the first non-zero digit, are not significant. These zeros merely serve as placeholders and do not contribute to the precision of the number. For example, in the number 0.00123, the leading zeros are not significant.

Trailing zeros, or zeros that appear after the last non-zero digit, are significant if they are to the right of a decimal point. These zeros indicate the precision of the measurement. For instance, in the number 123.4500, the trailing zeros are significant because they are to the right of a decimal point.

By following these rules, you can accurately determine the number of significant figures in a number. This ensures that your measurements and calculations reflect the true precision of your data, preventing the over-interpretation of results.

Rules for Counting Significant Figures

Counting significant figures may seem like a simple task, but it requires an understanding of specific rules to ensure accuracy. These rules help identify which digits in a number are significant and contribute to its precision. By following these guidelines, you can ensure that your measurements and calculations are as precise as your data allows.

The first rule for counting significant figures is that all non-zero digits are significant. These digits always contribute to the precision of the number. For example, in the number 456.78, all five digits are significant because they are non-zero.

The second rule is that any zeros between non-zero digits are significant. These zeros serve as placeholders and indicate the precision of the measurement. For instance, in the number 407.09, the zeros are significant because they fall between non-zero digits.

Leading zeros, or zeros that appear before the first non-zero digit, are not significant. These zeros merely serve as placeholders and do not contribute to the precision of the number. For example, in the number 0.00045, the leading zeros are not significant.

Trailing zeros, or zeros that appear after the last non-zero digit, are significant if they are to the right of a decimal point. These zeros indicate the precision of the measurement. For instance, in the number 89.6700, the trailing zeros are significant because they are to the right of a decimal point.

By adhering to these rules, you can accurately count the number of significant figures in a number. This ensures that your measurements and calculations reflect the true precision of your data, preventing the misinterpretation of results.

How Many Sig Figs in 0.1?

The question of how many significant figures are in the number 0.1 is a common one, and the answer is straightforward: there is one significant figure in 0.1. The number "1" is the only significant digit, as it contributes to the precision of the number. The zero before the decimal point is not counted as significant because it merely serves as a placeholder, indicating the position of the decimal.

Understanding why there is only one significant figure in 0.1 requires a grasp of the basic rules of significant figures. As mentioned earlier, leading zeros are not considered significant because they do not contribute to the precision of the measurement. In 0.1, the zero is a leading zero, and thus, it is not significant.

The significance of recognizing the number of significant figures in 0.1 extends beyond just counting digits. It also influences how calculations are performed. When using 0.1 in mathematical operations, the precision of the result is limited by the one significant figure in 0.1. This ensures that the final result accurately reflects the precision of the input data.

In summary, 0.1 has one significant figure, represented by the digit "1." Understanding this is crucial for accurately interpreting and using the number in calculations, ensuring that the precision of the result aligns with the precision of the input data.

Significant Figures in Scientific Notation

Scientific notation is a powerful tool for expressing very large or very small numbers in a concise and precise manner. In scientific notation, numbers are expressed as a product of a coefficient and a power of ten. The coefficient in scientific notation contains significant figures, while the power of ten indicates the scale of the number.

Understanding significant figures in scientific notation is crucial because it ensures that the precision of the number is maintained. For example, the number 0.1 can be expressed in scientific notation as 1.0 x 10^-1. In this case, the coefficient "1.0" has two significant figures, indicating the precision of the number. The power of ten, "-1," indicates the scale of the number but does not contribute to its precision.

When working with scientific notation, it's important to apply the rules of significant figures to the coefficient. This ensures that the precision of the number is accurately represented. For instance, in the number 3.45 x 10⁴, the coefficient "3.45" has three significant figures, indicating the precision of the number.

In summary, significant figures play a crucial role in scientific notation by ensuring that the precision of the number is accurately represented. The coefficient in scientific notation contains significant figures, while the power of ten indicates the scale of the number. By applying the rules of significant figures to scientific notation, you can ensure that your results accurately reflect the precision of your data.

Rounding and Significant Figures

Rounding is a common practice used to simplify numbers, making them easier to work with. However, when rounding numbers, it's important to consider significant figures to ensure that the precision of the data is maintained. Rounding without considering significant figures can lead to inaccurate results and misinterpretation of data.

The process of rounding involves reducing the number of digits in a number while maintaining its overall value and precision. When rounding to a specific number of significant figures, it's essential to follow specific rules to ensure accuracy. For example, if you're rounding the number 123.456 to three significant figures, the result would be 123. The number is rounded to the nearest significant digit, ensuring that the precision of the data is maintained.

One important rule to consider when rounding is that if the digit to be removed is greater than or equal to 5, the preceding digit is increased by one. For example, if you're rounding the number 3.678 to three significant figures, the result would be 3.68. The digit "8" is greater than or equal to 5, so the preceding digit "7" is increased by one.

By following these rules and considering significant figures when rounding, you can ensure that your results accurately reflect the precision of your data. This is crucial for maintaining the integrity of scientific research and ensuring that conclusions are drawn based on accurate information.

Significant Figures in Calculations

When performing calculations, it's important to consider significant figures to ensure that the precision of the results is maintained. The rules of significant figures play a crucial role in determining the precision of the final result, ensuring that it accurately reflects the precision of the input data.

In multiplication and division, the result should have the same number of significant figures as the measurement with the fewest significant figures. For example, if you're multiplying 3.45 (three significant figures) by 2.1 (two significant figures), the result should have two significant figures. The calculation would be 3.45 x 2.1 = 7.245, rounded to 7.2.

In addition and subtraction, the result should have the same number of decimal places as the measurement with the fewest decimal places. For example, if you're adding 12.34 (two decimal places) and 1.2 (one decimal place), the result should have one decimal place. The calculation would be 12.34 + 1.2 = 13.54, rounded to 13.5.

By following these rules, you can ensure that your calculations accurately reflect the precision of the input data. This is crucial for maintaining the integrity of scientific research and ensuring that conclusions are drawn based on accurate information.

Common Mistakes and Misconceptions

When working with significant figures, it's easy to make mistakes or fall into common misconceptions. Understanding these common pitfalls can help you avoid errors and ensure that your data is accurately represented.

One common mistake is confusing significant figures with decimal places. While decimal places refer to the number of digits to the right of the decimal point, significant figures refer to the meaningful digits in a number. It's important to understand this distinction to ensure that your data is accurately represented.

Another common misconception is assuming that all zeros are insignificant. While leading zeros are not significant, trailing zeros to the right of a decimal point are significant. These zeros indicate the precision of the measurement and should be considered when determining significant figures.

It's also important to avoid rounding numbers too early in calculations. Rounding should be done at the end of the calculation to ensure that the precision of the data is maintained. Rounding too early can lead to inaccurate results and misinterpretation of data.

By understanding these common mistakes and misconceptions, you can ensure that your data is accurately represented and that your results reflect the true precision of your measurements.

Practical Applications of Significant Figures

Significant figures have a wide range of practical applications in various fields, from scientific research to engineering and beyond. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

In scientific research, significant figures play a crucial role in expressing the precision of measurements. They help communicate the level of certainty that accompanies those measurements, ensuring that results are stated as precisely as the data allows. This is essential for maintaining the integrity of scientific research and ensuring that conclusions are drawn based on accurate information.

In engineering, significant figures are used to express the precision of measurements and calculations. They help ensure that designs and projects are executed with the highest level of accuracy, preventing errors and ensuring the safety and reliability of structures and systems.

In everyday life, significant figures are used in various applications, from financial calculations to cooking and beyond. Understanding and applying the rules of significant figures can help ensure that results are accurate and reliable, leading to better decision-making and improved outcomes.

In summary, significant figures have a wide range of practical applications in various fields. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

Significant Figures in Different Fields

Significant figures are used in various fields to express the precision of measurements and calculations. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

In the field of chemistry, significant figures are used to express the precision of measurements and calculations. They help ensure that results are accurate and reliable, leading to better decision-making and improved outcomes. For example, when measuring the concentration of a solution, significant figures are used to express the precision of the measurement, ensuring that the result accurately reflects the true concentration.

In the field of physics, significant figures are used to express the precision of measurements and calculations. They help ensure that results are accurate and reliable, leading to better decision-making and improved outcomes. For example, when measuring the speed of an object, significant figures are used to express the precision of the measurement, ensuring that the result accurately reflects the true speed.

In the field of engineering, significant figures are used to express the precision of measurements and calculations. They help ensure that designs and projects are executed with the highest level of accuracy, preventing errors and ensuring the safety and reliability of structures and systems. For example, when designing a bridge, significant figures are used to express the precision of the measurements and calculations, ensuring that the design is accurate and reliable.

In summary, significant figures are used in various fields to express the precision of measurements and calculations. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

Real-World Examples

Significant figures play a crucial role in various real-world applications, from scientific research to engineering and beyond. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

In the pharmaceutical industry, significant figures are used to express the precision of measurements and calculations. They help ensure that dosages are accurate and reliable, preventing errors and ensuring patient safety. For example, when measuring the amount of active ingredient in a medication, significant figures are used to express the precision of the measurement, ensuring that the dosage is accurate and safe for patients.

In the field of construction, significant figures are used to express the precision of measurements and calculations. They help ensure that designs and projects are executed with the highest level of accuracy, preventing errors and ensuring the safety and reliability of structures and systems. For example, when measuring the dimensions of a building, significant figures are used to express the precision of the measurements, ensuring that the design is accurate and reliable.

In the field of finance, significant figures are used to express the precision of calculations. They help ensure that financial decisions are based on accurate and reliable data, leading to better decision-making and improved outcomes. For example, when calculating interest rates or investment returns, significant figures are used to express the precision of the calculations, ensuring that the results are accurate and reliable.

In summary, significant figures play a crucial role in various real-world applications. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information.

Frequently Asked Questions

1. What are significant figures?

Significant figures are the digits in a number that contribute to its precision. They are used in expressing numbers consistently in scientific and engineering contexts to indicate the reliability of measurements.

2. How do you determine the number of significant figures in a number?

To determine the number of significant figures, identify all non-zero digits as significant, any zeros between non-zero digits, and trailing zeros to the right of a decimal point. Leading zeros are not considered significant.

3. Why are significant figures important?

Significant figures are important because they help express the precision of measurements and calculations, ensuring that the results accurately reflect the true precision of the data.

4. How many significant figures are in the number 0.1?

The number 0.1 has one significant figure, represented by the digit "1." The zero before the decimal point is not counted as significant because it is a placeholder.

5. How do significant figures affect calculations?

In calculations, the result should have the same number of significant figures as the measurement with the fewest significant figures, ensuring that the precision of the result aligns with the precision of the input data.

6. What are some common mistakes when working with significant figures?

Common mistakes include confusing significant figures with decimal places, assuming all zeros are insignificant, and rounding numbers too early in calculations.

Conclusion

In conclusion, significant figures are a fundamental concept in mathematics, science, and engineering, playing a crucial role in expressing the precision of measurements and calculations. Understanding and applying the rules of significant figures is essential for ensuring that data is accurately represented and that conclusions are drawn based on precise information. By following the guidelines outlined in this article, you can ensure that your measurements and calculations reflect the true precision of your data, preventing the misinterpretation of results and maintaining the integrity of scientific research and engineering projects.

Further Reading and Resources

For those interested in delving deeper into the topic of significant figures, there are many resources available to enhance your understanding. Recommended reading includes textbooks on mathematics and science that cover significant figures in detail, as well as online resources that provide interactive tutorials and exercises. Additionally, consulting authoritative sources such as scientific journals and publications can provide valuable insights into the practical applications of significant figures in various fields.